Chapter 16 (cont..12)

The following solved example illustrates the design of a transverse stair.

Solved example 16.3

The reinforcement details according to the above solved example is shown in the figs.16.64 and 16.65 given below:

Fig.16.64
Sectional elevation of transverse stair

Fig.16.65
Section XX

We will now discuss the various features of the above two figs:
The main bars are given as the bottom most layer. The distributor bars are given as the second layer from the bottom. This arrangement will give maximum possible effective depth ‘d’ for the section. From the section XX, we can see that the main bars are given two 90^o bends at both the ends. This will give a 'hook' like arrangement at both ends. The top portion of these 'hooks' will act as the steel required for resisting any possible hogging moment at the supports. The length required for this top steel is 0.15l, where l is the effective span of the waist slab. But the area required for this top steel is only half of that at the mid span. So, as shown in the inset, only alternate bars are given these hooks.

The distributor bars are of mild steel. So the symbol Φ is given to denote them.

In the above example, only a part plan (shown in the fig.16.65 below) of the stair was given along with the problem data.

Fig.16.65
Problem data of solved example 16.3

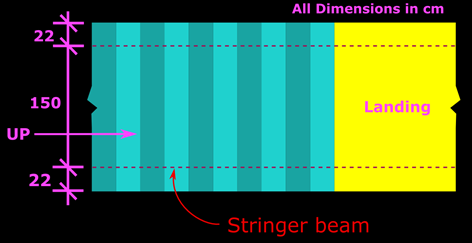
This is because, this small portion is sufficient for defining the problem of this type of a transverse stair. Some times this stair may have a landing also as shown below.

Fig.16.66
Landing in a transverse stair

In this case also the sloping portion can be analysed and designed by the same procedure, and the landing can be analysed and designed as a simply supported one-way slab.

Stairs cantilevering from the side of a beam:

The following fig.16.67 shows the part view of a stair.

Fig.16.67
View of a cantilever stair

It is projecting from the side of a wall. The wall is shown in a finished state. So we cannot see more details. The fig. below shows the view before the plastering is applied to the wall.

Fig.16.68
View of a stair cantilevering from a stringer beam

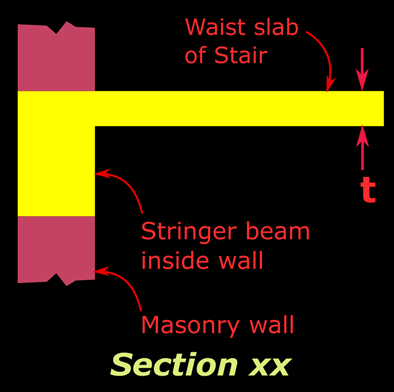
We can see that the stair is projecting from the side of a beam, which is concealed inside the wall. In actual construction, the beam (which frames into columns) and the waist slab will be cast first, and after curing and removal of form works, the masonry wall will be constructed above and below the beam. The elevation and section are shown in the figs. below:

Fig.16.69
Part elevation view of cantilever stairs

Fig.16.70
Section XX

The waist slab bends in a direction perpendicular to the direction of travel of the pedestrians, and so it is a transverse stair. The load calculation procedure is the same as that for the transverse stair supported on two stringer beams. So we can use the same Eq.16.25. The line diagram for the analysis will be as shown below:

Fig.16.80
Line diagram for cantilever stairs

The effective span l of the cantilever (cl.22.2.c) is the length of the cantilever up to the face of the support plus half the effective depth. For initial proportioning, we can assume the thickness of waist slab to be clear length of cantilever/10 . However, this should be finalized only after the complete design and doing the various checks.

In the next section, we will see a solved example of the above type of stairs.

PREVIOUS      CONTENTS      NEXT

             Copyright ©2015 limitstatelessons.blogspot.com - All Rights Reserved