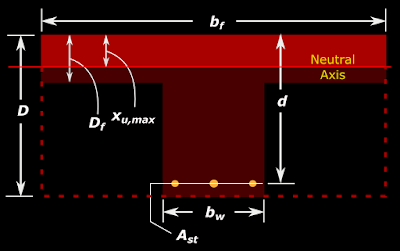
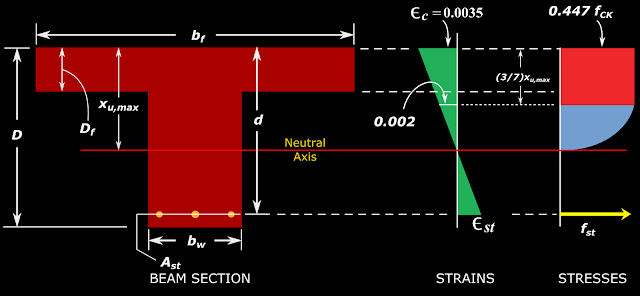
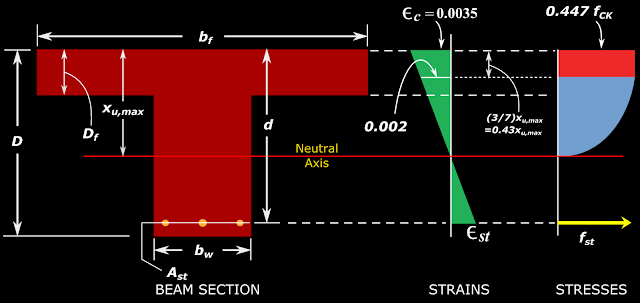
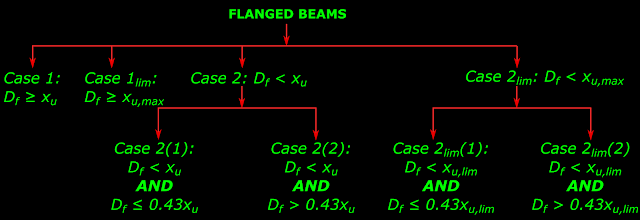
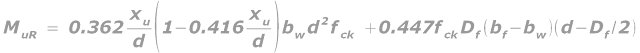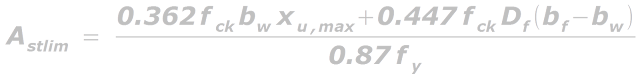In the previous section, we have discussed the method for calculating the Mulim of any given flanged section. Now we will calculate the Mulim of the sections in each of the solved examples that we did in this chapter:
Solved example 9.1:
The data for this example are:
bf =950 mm, Df =110 mm, D =600 mm, bw =300 mm, d =520 mm, Ast =3694.51 mm2 (6-28Ф), fy = 250 N/mm2, fck =20N/mm2
Calculations:
For Fe250 steel, xu,max / d = 0.531 (Table 3.4)
So xu,max for our beam section = 0.531 x 520 =276.12
Here xu,max > Df so the section belongs to either case 2lim(1) or case 2lim(2)
0.43xu,max = 0.43 x 276.12 = 118.8 > Df. So the beam belongs to case 2lim(1)
So we can use Eq.9.11
• Change xu to xu,max.
• Change MuR to Mulim
Substituting all the known values, we will get Mulim = 540.3 kNm
We can calculate Ast,lim using
Eq.9.19
Substituting all the known values, we get Ast,lim = 5697.85 mm2.
Let us compare the above results with those of the solved example 9.1:
The results obtained in 9.1 were:
[xu = 127.50 mm] < [xu,max = 276.12 mm] Hence under reinforced
[MuR = 379.3 kNm] < [Mulim =540.3 kNm] as expected
[Ast = 3694.51 mm2] < [Ast,lim =5697.85 mm2] as expected.
Solved example 9.2:
The data for this example are:
bf =1300 mm, Df =100 mm, D =500 mm, bw =325 mm, d =420 mm, Ast =4310.27 mm2 (7-28Ф), fy = 250 N/mm2, fck =20N/mm2
Calculations:
For Fe415 steel, xu,max / d = 0.4791 (Table 3.4)
So xu,max for our beam section = 0.4791 x 420 =201.22 mm
Here xu,max > Df so the section belongs to either case 2lim(1) or case 2lim(2)
0.43xu,max = 0.43 x 201.22 = 86.53 < Df. So the beam belongs to case 2lim(2)
So we can use Eq.9.17
• Change xu to xu,max.
• Change MuR to Mulim
Substituting all the known values, we will get Mulim = 468.2 kNm
We can calculate Ast,lim using
Eq.9.19
• Change Df to yf
Substituting all the known values, we get Ast,lim = 3609.3 mm2.
Let us compare the above results with those of the solved example 9.2:
The results obtained in 9.2 were:
[xu = 260.51 mm] > [xu,max = 201.22 mm] Hence over reinforced
[MuR = 509.45 kNm] > [Mulim =468.2 kNm] as expected
[Ast = 4310.27 mm2] > [Ast,lim =3609.3 mm2] as expected.
Solved example 9.3:
The data for this example are:
bf =1000 mm, Df =100 mm, D =500 mm, bw =325 mm, d =420 mm, Ast =3436.12 mm2 (7-25Ф), fy = 415 N/mm2, fck =25 N/mm2
Calculations:
For Fe415 steel, xu,max / d = 0.4791 (Table 3.4)
So xu,max for our beam section = 0.4791 x 420 =201.22 mm
Here xu,max > Df so the section belongs to either case 2lim(1) or case 2lim(2)
0.43xu,max = 0.43 x 201.22 = 86.53 < Df. So the beam belongs to case 2lim(2)
So we can use Eq.9.17
• Change xu to xu,max.
• Change MuR to Mulim
Substituting all the known values, we will get Mulim = 466.41 kNm
We can calculate Ast,lim using
Eq.9.19
• Change Df to yf
Substituting all the known values, we get Ast,lim = 3627.82 mm2.
Let us compare the above results with those of the solved example 9.3:
The results obtained in 9.3 were:
[xu = 184.23 mm] < [xu,max = 201.22 mm] Hence under reinforced
[MuR = 447.16 kNm] < [Mulim =466.41 kNm] as expected
[Ast = 3436.12 mm2] < [Ast,lim =3627.82 mm2] as expected.
Solved example 9.4:
The data for this example are:
bf =1532 mm, Df =110 mm, D =510 mm, bw =250 mm, d =464 mm, Ast =603.19 mm2 (3-16Ф)., fy = 415 N/mm2, fck =20 N/mm2
Calculations:
For Fe415 steel, xu,max / d = 0.4791 (Table 3.4)
So xu,max for our beam section = 0.4791 x 464 =222.30 mm
Here xu,max > Df so the section belongs to either case 2lim(1) or case 2lim(2)
0.43xu,max = 0.43 x 222.3 = 95.59 < Df. So the beam belongs to case 2lim(2)
So we can use Eq.9.17
• Change xu to xu,max.
• Change MuR to Mulim
Substituting all the known values, we will get Mulim = 644.05 kNm
We can calculate Ast,lim using
Eq.9.19
• Change Df to yf
Substituting all the known values, we get Ast,lim = 4442.61 mm2.
Let us compare the above results with those of the solved example 9.4:
The results obtained in 9.4 were:
[xu = 19.635 mm] < [xu,max = 222.30 mm] Hence under reinforced
[MuR = 99.27 kNm] < [Mulim =644.05 kNm] as expected
[Ast = 603.19 mm2] < [Ast,lim =4442.61 mm2] as expected.
With this we have completed the discussion on the analysis of flanged sections. In the next section we will see the design part.
PREVIOUS
Solved example 9.1:
The data for this example are:
bf =950 mm, Df =110 mm, D =600 mm, bw =300 mm, d =520 mm, Ast =3694.51 mm2 (6-28Ф), fy = 250 N/mm2, fck =20N/mm2
Calculations:
For Fe250 steel, xu,max / d = 0.531 (Table 3.4)
So xu,max for our beam section = 0.531 x 520 =276.12
Here xu,max > Df so the section belongs to either case 2lim(1) or case 2lim(2)
0.43xu,max = 0.43 x 276.12 = 118.8 > Df. So the beam belongs to case 2lim(1)
So we can use Eq.9.11
• Change xu to xu,max.
• Change MuR to Mulim
Substituting all the known values, we will get Mulim = 540.3 kNm
We can calculate Ast,lim using
Eq.9.19
Substituting all the known values, we get Ast,lim = 5697.85 mm2.
Let us compare the above results with those of the solved example 9.1:
The results obtained in 9.1 were:
[xu = 127.50 mm] < [xu,max = 276.12 mm] Hence under reinforced
[MuR = 379.3 kNm] < [Mulim =540.3 kNm] as expected
[Ast = 3694.51 mm2] < [Ast,lim =5697.85 mm2] as expected.
Solved example 9.2:
The data for this example are:
bf =1300 mm, Df =100 mm, D =500 mm, bw =325 mm, d =420 mm, Ast =4310.27 mm2 (7-28Ф), fy = 250 N/mm2, fck =20N/mm2
Calculations:
For Fe415 steel, xu,max / d = 0.4791 (Table 3.4)
So xu,max for our beam section = 0.4791 x 420 =201.22 mm
Here xu,max > Df so the section belongs to either case 2lim(1) or case 2lim(2)
0.43xu,max = 0.43 x 201.22 = 86.53 < Df. So the beam belongs to case 2lim(2)
So we can use Eq.9.17
• Change xu to xu,max.
• Change MuR to Mulim
Substituting all the known values, we will get Mulim = 468.2 kNm
We can calculate Ast,lim using
Eq.9.19
• Change Df to yf
Substituting all the known values, we get Ast,lim = 3609.3 mm2.
Let us compare the above results with those of the solved example 9.2:
The results obtained in 9.2 were:
[xu = 260.51 mm] > [xu,max = 201.22 mm] Hence over reinforced
[MuR = 509.45 kNm] > [Mulim =468.2 kNm] as expected
[Ast = 4310.27 mm2] > [Ast,lim =3609.3 mm2] as expected.
Solved example 9.3:
The data for this example are:
bf =1000 mm, Df =100 mm, D =500 mm, bw =325 mm, d =420 mm, Ast =3436.12 mm2 (7-25Ф), fy = 415 N/mm2, fck =25 N/mm2
Calculations:
For Fe415 steel, xu,max / d = 0.4791 (Table 3.4)
So xu,max for our beam section = 0.4791 x 420 =201.22 mm
Here xu,max > Df so the section belongs to either case 2lim(1) or case 2lim(2)
0.43xu,max = 0.43 x 201.22 = 86.53 < Df. So the beam belongs to case 2lim(2)
So we can use Eq.9.17
• Change xu to xu,max.
• Change MuR to Mulim
Substituting all the known values, we will get Mulim = 466.41 kNm
We can calculate Ast,lim using
Eq.9.19
• Change Df to yf
Substituting all the known values, we get Ast,lim = 3627.82 mm2.
Let us compare the above results with those of the solved example 9.3:
The results obtained in 9.3 were:
[xu = 184.23 mm] < [xu,max = 201.22 mm] Hence under reinforced
[MuR = 447.16 kNm] < [Mulim =466.41 kNm] as expected
[Ast = 3436.12 mm2] < [Ast,lim =3627.82 mm2] as expected.
Solved example 9.4:
The data for this example are:
bf =1532 mm, Df =110 mm, D =510 mm, bw =250 mm, d =464 mm, Ast =603.19 mm2 (3-16Ф)., fy = 415 N/mm2, fck =20 N/mm2
Calculations:
For Fe415 steel, xu,max / d = 0.4791 (Table 3.4)
So xu,max for our beam section = 0.4791 x 464 =222.30 mm
Here xu,max > Df so the section belongs to either case 2lim(1) or case 2lim(2)
0.43xu,max = 0.43 x 222.3 = 95.59 < Df. So the beam belongs to case 2lim(2)
So we can use Eq.9.17
• Change xu to xu,max.
• Change MuR to Mulim
Substituting all the known values, we will get Mulim = 644.05 kNm
We can calculate Ast,lim using
Eq.9.19
• Change Df to yf
Substituting all the known values, we get Ast,lim = 4442.61 mm2.
Let us compare the above results with those of the solved example 9.4:
The results obtained in 9.4 were:
[xu = 19.635 mm] < [xu,max = 222.30 mm] Hence under reinforced
[MuR = 99.27 kNm] < [Mulim =644.05 kNm] as expected
[Ast = 603.19 mm2] < [Ast,lim =4442.61 mm2] as expected.
With this we have completed the discussion on the analysis of flanged sections. In the next section we will see the design part.
PREVIOUS