In the previous section we were discussing the sub clauses which gives the specifications for 'increasing lap length based on concrete cover'. In this section, we continue the discussion.
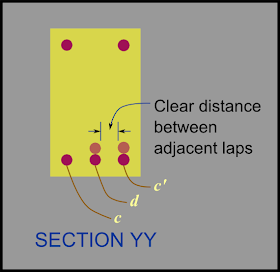
The sub clause (2), specifies another condition about ‘adjacent laps’. For discussing this, let us assume that both the bars c' and d are lap spliced at the same section. Then the section YY will be as shown below:Fig.14.56
Section YY when laps are present in adjacent bars
In this YY section, the clear distance between the adjacent laps is shown. If this clear distance is less than the largest of 75 mm and 6Ф, then we have an example of the other condition about ‘adjacent laps’, specified in this sub clause (2). In this case also we must take the same steps as before:
• First, find L by following the 'rules for obtaining the lap length for bars in flexural tension' that we discussed above. (Figs.14.48 to 14.50).
• Then obtain the modified value by multiplying this L by 1.4.
This modified value should be provided for the lap. Note that this condition involving the distance between adjacent laps is applicable to both top bars and bottom bars.
So in general, if a bar is such that, only the sub clause (2) is applicable (for computing required increments in lap lengths based on covers), then any one of the two points below should be satisfied by the bar:
■ It should be a corner bar
♦ Any of ct /cb OR cs should cause concern.
■ It should be one among two adjacent bars which are lapped at the same section, with a clear distance less than the largest of 75 mm and 6Ф.
So we now know how to determine if a bar falls into sub clauses (1) or (2). At the end of sub clause (2), one more condition is specified. That is., if a bar falls into both the sub clauses (1) and (2), then the multiplication factor to be used is '2' instead of '1.4'.
Let us now see whether this condition is applicable to any of the bars in the above continuous beam. In the section XX, consider the bars a and a'. For them, ct causes concern. If this ct is less than 2Ф, then they will fall into (1). For these bars cs also causes concern (∵ the beam is not a T-beam). If this cs is less than 2Ф, then they will fall into (2) also. If they fall into both (1) and (2), the multiplication factor to be used for them is '2' instead of '1.4'. If it is a T-beam, cs will not cause concern, and so (2) will not be applicable. Then the multiplication factor will remain as '1.4'.
Now consider section ZZ. All the top bars e, e' and d will be in tension. So for them, ct causes concern. If this ct is less than 2Ф, then they will fall into (1). The bars e and e', besides being top tension bars, are corner bars also. For them cs also cause concern. If this cs is less than 2Ф, then they will fall into (2) also. If they fall into both (1) and (2), the multiplication factor to be used for them is '2' instead of '1.4'.
But in the above fig, the top bars are all bent, and extended into the column. So in the column portion, they are having a cover from the face of the column. If this cover is less than twice the diameter, then bar d will be in such a position that, both the conditions are applicable to it also. So in that case, the modification factor for bar d will also be '2'. For these bars, another possibility is that the top bars have the specified cover from the top portion of the beam, but do not have it from the side of the column. Then also the modification factor should be '2'.
What we saw above are only a few examples of the bars for which the cl.26.2.5.1(c) and it’s sub clauses may be applicable. The designer should carefully examine each structural member to determine the clauses which are applicable to the different bars in it. Also, any type of splicing should be avoided at regions of high stresses, as we discussed earlier.
So we have had a lengthy discussion about the sub clause (c) and the sub clauses (1) and (2) within it. It is convenient to show an abstract of the discussion in the form of a flow chart as shown below:
So we have completed the discussion about all the specifications regarding the 'length of the lap'. It is time to see the specification about 'Staggering of laps'. This is given in the cl.26.2.5.1(b). According to this clause, the lap splices can be considered to be staggered if the centre to centre distance between the lap splices is not less than 1.3 times the lap length L. This is shown in the fig.14.57 given below.
Fig.14.57
Staggering of lapped splices
Note that for this clause to be applicable, L should be calculated exactly according to the guidelines given in the cl.26.2.5.1(c), and it's sub clauses (1) and (2), which we discussed above.
Cl.26.2.5.1(d)
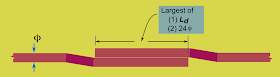
This clause is about the lap length required in compression. According to this clause, the lap length in compression should not be less than the largest of the following: (1) Development length Ld of the bar in compression, and (2) 24Ф. We can make a fig similar to the one we used to show the lap length for bars in tension. Such a fig is shown below:
Fig.14.58
Lap length in compression
We have to take special care about the item (1), the Ld in compression. For a bar of a particular diameter, Ld in compression will be different from Ld in tension. We have already discussed how to calculate Ld in compression. See the notes given below Table 14.1 and the example of a doubly reinforced cantilever beam shown in fig.14.5
When bends or hooks are provided at the lapped ends, only their 'projected length' can be considered for calculating the Ld. We discussed about this based on fig.14.16 in the section: 'Bends and hooks for compression reinforcement'. If bends and hooks are provided at the lapped ends of bars in compression, it is better to ignore their projected length, and consider the straight portion only. This is shown in the figs. below.
Fig.14.59
Lap length in compression when hooks are provided
Fig.14.60
Lap length in compression when bends are provided
It may be noted that for determining the lap length for bars in tension, the code gives different methods for ‘flexural tension’ and ‘direct tension’. But there is no such differentiation for the laps of bars in compression.
Cl.26.2.5.1(e)
This clause is about the lapping of two bars having different diameters. We have seen that Ф, the diameter of the bar comes into the calculation of development length Ld, Lap length L, etc., This clause tells us to use the smaller Ф in the calculations, when bars of two diameters have to be lap spliced.
Cl.26.2.5.1(f)
This clause is about the splicing of welded wire fabric.
Cl.26.2.5.1(g)
This clause is about the splicing of bars within a bundle. Bundles as a whole should not be spliced. If the bars of a bundle fall short of length, they can be lap spliced one bar at a time. Care should be taken to see that these splices are staggered, and are not at sections of maximum stress. The modification factors that we saw earlier in sub clause (c) should be applied where ever necessary. Also, the increase in development lengths to be applied particularly for bars in a bundle, that we discussed based on fig.14.9 should also be taken into account.
This completes the discussion about lapped splices. So we will now see some basic details about welded splices and mechanical connections.
For large diameter bars, welded splices and mechanical connections are more suitable. When these methods are adopted, extra length of steel bars for the lapping is not required. So it will result in a lower consumption of steel. Cl.12.4 of the code gives the recommendations regarding welded joints or mechanical connections. According to this clause, tests must be conducted on the bars spliced by these methods, to ensure that they have the full strength as the bars which are connected. Cl.26.2.5.2 gives the value of the strength of the weld. According to this clause, if the tests prove that the weld in a bar used as tensile reinforcement, has the same strength as the parent bar, then the design should be in such a way that, only 80 per cent of the full design strength of the bar will be applied on that welded bar.
More details about welded splices and mechanical connections, and the details of tests that have to be performed, can be obtained from the relevant codes.
So we have completed the discussion on splices. The next chapter discusses the details about 'Curtailment of bars'.
Copyright©2016 limitstatelessons.blogspot.com- All Rights Reserved




No comments:
Post a Comment