In the previous section we were discussing the method of curtailment when Moment coefficients are used for the analysis of continuous beams. In this section, we continue the discussion.
Fig.15.86
Arrangement after curtailment
In this case, there are only three bars. Only one of them can be curtailed because two bars have to continue as stirrup hangers. So there will be only one stage of curtailment. The calculations are shown in the fig. below:
Fig.15.87
Calculations of areas after curtailment
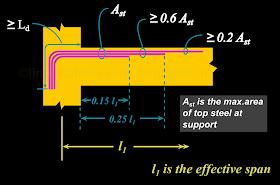
We can see that the two 16 mm dia. bars give an area of 66.67%. This is greater than the required 60%. So the middle 16 mm bar can be curtailed at 0.15 l1. There is no need to take it upto 0.25 l1. However, the above arrangement should be finalized only after the development length checks using the values of l1 and l2
Let us consider one more example in which 2-16# and 1-20# are given. In this case also, there can be only one stage of curtailment, and that is of the 20 mm dia. bar. The two 16 mm dia. bars have to continue as stirrup hangers. The calculations are given below:
Fig.15.88
Calculations of areas after curtailment
We can see that after curtailment, only 56.14% remains. But we want 60%. So we must extend the bar up to 0.25 l1. In other words, the curtailment should be done only at 0.25 l1. And also, the development length checks should be done using the values of l1 and l2 . The arrangement is shown below:
Fig.15.89
Arrangement after curtailment
So we have completed the discussion about the curtailment of top bars when 'Moment coefficients are used for design of steel'. But in the discussions, we did not consider the case when the end support of the continuous beam is simply supported. In such a support condition, there will not be any bending moment, and the arrangement shown in fig.15.58 that we discussed earlier can be provided.
Now we will discuss about the bottom bars. We know that when Moment coefficients are used, the sagging BM at midspans are also calculated using coefficients. So there will not be any BM diagrams, and we will have to follow the same fig.8.15 of SP 34.
Fig.15.90
Curtailment of bottom bars when Moment coefficients are used
From the fig.15.90, we can see that there is only one stage of curtailment for the bottom bars. The distance of this curtailment is 0.1 l at end supports, and 0.15 l1 at intermediate supports. These distances are measured from the 'imaginary vertical lines within the supports' which mark the effective spans. The area of remaining bars which continue towards the supports is same for both end supports and continuous supports, and is equal to 0.3Ast .
We can work out a quick example to demonstrate the area requirements. Let there be 3-16# as bottom bars in a beam. The middle one can be curtailed. The other two has to continue because, there must be two corner bars at the bottom for the stirrups. So the percentage of 2-16# is equal to 66.67. (calculations are same as that in fig.15.87 above) This is greater than the required 30%.
Let us see another example with 2-16# + 1-20# . The middle 20 mm bar can be curtailed. So the percentage of 2-16# is equal to 56.14. (calculations are same as that in fig.15.88 above) This is greater than the required 30%.
So we will get the curtailment details of ‘bottom bars within the span’ from the above fig.15.90. But we have to work out the following separately:
(i) amount of bottom bars that has to be embedded in the supports.
(ii) length of bottom bars that has to be embedded in the supports.
(iii) Development length requirements for bottom bars at supports.
(i) and (ii) above were discussed before, when we saw the details about cl.26.2.3.3(a) of the code. We saw the figs.15.42 to 15.45 in chapter 15.9, which are based on this clause. So, using those figs., we can calculate (i) and (ii) .
(iii) was also discussed before. The procedure for both simple supports and continuous supports were discussed. MuR is the ‘Ultimate moment of resistance’ of the section, and Vu is the factored shear force at the support. The shear forces are determined by using the coefficients from Table 13.
Thus we are now in a position to do the curtailment design of a continuous beam when it is designed using ‘Moment coefficients’. The very same procedure can be used also for a continuous slab, when it is designed using moment coefficients. For this we must use fig.9.5 of SP 34.
Curtailment of Bundled bars
When bundled bars are to be curtailed, all the bars in the bundle should not be curtailed at a single section. Each bar must be curtailed at sections which are at least 40Φ apart. This is shown in the fig.15.85 below:
Fig.15.91
Curtailment of individual bars in a bundle
First, the whole bundle is given an extension of La beyond the theoretical cut-off point. Then the individual members of the bundle are curtailed one at a time, and the distance between the sections are greater than or equal to 40Φ. [In the fig., the bars of the bundle are shown separately. This is only for clarity. In actual case, all the bars of a bundle will be in contact]
Another point to note is that while curtailing bundled bars, the bars which are closer to the Neutral axis should be curtailed first. So for a bundled bar at the top of a beam, the bars which are at the bottom in the bundle should be curtailed first. Similarly, for a bundled bar at the bottom of a beam, the bars which are at the top in the bundle should be curtailed first. This is shown in the figs. below:
Fig.15.92
Bundled bar at top of beam section
Fig.15.93
Bundled bar at bottom of beam section
After fixing up the final curtailments, we have to check for development lengths. This is necessary for bundled bars also. We have seen that this check is done based on fig.15.50 (bottom bars) and fig.15.60 (top bars). Now, while checking this for bundled bars, the increased value of Ld should be used based on fig.14.9. This increased value should be available on both sides of a section.
As an example, let us consider the curtailment involving a bundle of 3 nos. of 12 mm bars. (Assume that the bars are in tension, the bars are of grade Fe 415 deformed steel, and the grade of concrete is M20) From fig.14.9 we see that Ld of each bar of a bundle of 3 bars should be increased by 20%. We have also seen that for the above assumptions, Ld of 12 mm bars is equal to 564.14 mm. Increasing this by 20%, we get 564.14 x 1.2 = 676.97 = 677 mm.
• If our bundle belongs to the ‘category of curtailed bars’ in fig.15.50, then the bundle as a whole should have a distance greater than 677 mm on both sides of CL, and then only the curtailment of individual bars can begin. (The individual bars should be curtailed at sections which are more than 40 x 12 = 480 mm apart.)
• If it belongs to the ‘category of continuing bars’, then the distance on both sides of the theoretical cut-off section should be greater than 677 mm. These requirements are shown in the figs. below:
Fig.15.94
Development length requirements of a 'curtailed' bundled bar
Fig.15.95
Development length requirements of a 'continuing' bundled bar
So we have completed the discussion about the curtailment of bars. We will see some solved examples.
Solved example 15.1
In this example we will design the curtailment and layout of the bars of the beam that we designed in solved example 4.3
In that example, the final section was designed. Now, The design of curtailment and layout is given now as the Solved example 15.1
Solved example 15.2
In this example we will do the ‘shear design’ of the beam that we designed just above in solved example 15.1. While doing this, we will be considering the points that have to be checked while doing the shear design of a beam in which curtailment of tensile bars have been done.
In the next chapter we will discuss about the design of Stairs.
Copyright©2016 limitstatelessons.blogspot.com - All Rights Reserved