In the previous section we saw the details about 'bearing stress'. In this section we will discuss about splices.
Splicing of bars
Sometimes, a bar placed in a structural member will fall short of it's required length. In such a situation, the bar can be extended by splicing. When this is done, the axial force in the bar should be transferred effectively from the bar which fell short of length, to the new continuing bar. There are three methods for splicing.• Lap splice
• Welded splice
• Mechanical connection.
First we will see the details about Lap splice. In this method, the two bars to be spliced are overlapped over a certain distance. The lapped bars are placed in contact and tied together lightly, so that they stay in place when the concrete is placed and compacted. In this type of splice, the force in the first bar is first transferred to the concrete by the action of bond stress. From there, the force is transferred to the continuing bar, again by the action of bond stress.
Details of a model of a lapped splice which is used in situation other than Reinforced concrete work can be seen here.
It should be noted that, lap splicing is not permitted for bars having diameter greater than 36 mm. The following figs., give some details about lap splice.
Fig.14.32
Overlapping of bars
In the above fig., Bar A is the one which falls short of length. The end portion of this bar from A to D is shown in the fig. Bar B is the continuing bar. We can see that the centre lines of the two bars do not coincide. This will give rise to eccentricity. We will now see the procedure for avoiding this type of eccentricity at the region of overlapping. The next fig.14.33 shows a small deviation given to the bar AD.
Fig.14.33
Deviation to the bar
For making the deviation, the bar is given a small bend at B. The bend should be in such a way that, the slope of the portion BD is not greater than 1 in 6. In the fig, the slope of BD is given exactly equal to 1 in 6 as shown by the blue triangle. (The position of B at which we must give the bend, will be clear after a few more steps). Now the bar is deviating away from the original center line. So we give it one more bend, but this time, in the reverse direction. This is shown in the next fig.14.34
Fig.14.34
Bend in the portion BD
From the fig.14.34, we can see that the second bend is given at point C. This point lies at the intersection of the portion BD (in the previous fig. 14.33) with the center line. The bend at C should be given in such a way that the portion CD becomes parallel to the center line. When this is done, the whole portion from C to D lies just below the center line.
We can calculate the length BC from the similar triangles (colored in orange) in the fig.14.35 given below:
Fig.14.35
Calculation of length BC
From similar triangles in BCC’ we get
So BC’ = 3Ф
BC’ is the altitude, and C’C is the base of the right angled triangle BCC’ . So we get
From the above, we can see that the 'slope of the bend' and Ф, are the only two parameters that determine the length of BC.
So we have obtained the final shape of Bar A. Now, Bar B comes from the opposite side. So we will make a mirror image of Bar A, as shown below in fig.14.36. The mirror line is vertical. But the bent portion should be in the upper side. So we will make another mirror image with a horizontal mirror line. This gives the final shape of Bar B.
Fig.14.36
Final shape of Bar B
So this Bar B can be lapped onto Bar A as shown in the fig.14.37 below:
Fig.14.37
Lapped bars
When this is done, centre lines of both the bars will coincide, and thus there will not be any eccentricity. The over lapping length L shown in the fig.13.37 must be equal to the development length Ld of the bar.
We have seen in fig.14.33 a little while earlier that, the slope of portion BC should not be greater than 1 in 6. The fig.14.38 given below shows the same bar, but with a slope of 2 in 6.
Fig.14.38
Slope of BC greater than 1 in 6
We can see that when a greater slope is given, BC becomes steeper, and it’s length is reduced. This will not give an effective transfer of force. So any slope greater than 1 in 6 is not permitted.
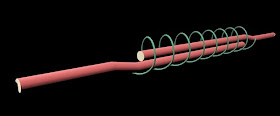
Now we will see a special case of bars with larger diameters. Lap splices should not be used for bars of diameter greater than 36 mm. For such bars, welded splices are recommended. But when welding is not practicable, lap splices can be used for such bars as per cl.26.2.5.1a of the code. According to this clause, such lap splices should be given additional spirals as shown in the fig.14.39 below.
Fig.14.39
Additional spirals at lap
The bars used for these spirals should have a minimum diameter of 6 mm, and the pitch should not be greater than 100 mm. This is shown in the elevation view of the above fig.
Fig.14.40
Maximum pitch and minimum diameter for spirals
In the next section we will see more details about lap splice.
Copyright©2016 limitstatelessons.blogspot.com - All Rights Reserved









It should be noted that, lap splicing is not permitted for bars having diameter greater than 36 mm......After amendment it is now 32mm
ReplyDeleteThanks for this information. Please post the details also. Date of publication of the amendment, Clause number, etc.,
Delete